
It has one more interesting property, which is related to the mean values of our observations. It isn't hard to note that the intercept coefficient b indicates the point on the vertical axis through which the fitted line passes. sd(x) is the standard deviation of x and.corr(x, y) is the correlation between x and y.Interestingly, we can express the slope a in terms of the standard deviations of x and y and of their Pearson correlation. If a = 0, then there is no relationship between the two variables in question: the value of y is the same (constant) for all values of x.We say there is a negative relationship between the two variables: as one increases, the other decreases. If a We say there is a positive relationship between the two variables: as one increases, the other increases as well. If a > 0, then y increases by a units whenever x increases by 1 unit.Indeed, let's take a look at the following simple calculation:Ī * (x + 1) + b = (a * x + b) + a = y + a. It describes how much the dependent variable y changes (on average!) when the incoefficient of determination**, dependent variable x changes by one unit**. The coefficient a is the slope of the regression line. A simple example is when we want to predict the weights of students based on their heights, or in chemistry, where linear regression is used in the calculation of the concentration of an unknown sample.īe careful, as in some situations simple linear regression may not be the right model! If your data seem to follow a parabola rather than a straight line, then you should try using our quadratic regression calculator, if they rather resemble a cubic (degree three) curve, try the cubic regression calculator, while if your data come from a process characterized by exponential growth, try the exponential regression calculator instead.
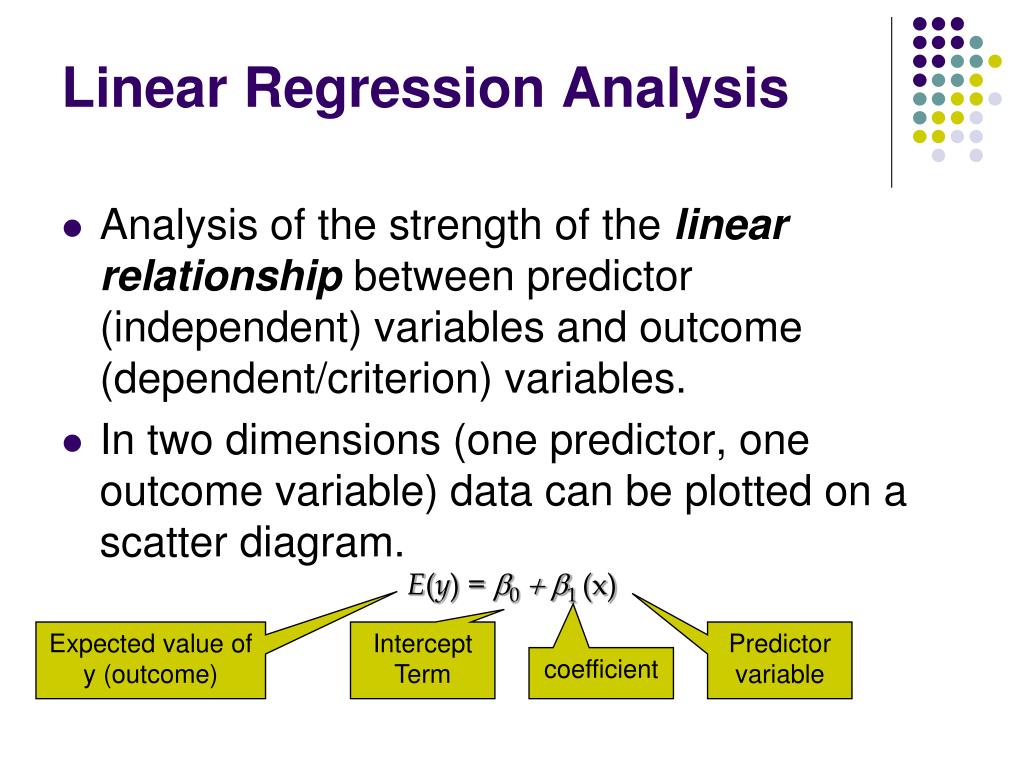
In other words, when we have a set of two-dimensional data points, linear regression describes the (non-vertical) straight line that best fits these points. Linear regression is a statistical technique that aims to model the relationship between two variables (one variable is called explanatory/independent and the other is dependent) by determining a linear equation that best predicts the values of the dependent variable based on the values of the independent variable.


 0 kommentar(er)
0 kommentar(er)
